Spur Gear Terminology and Calculation

July 19, 2024 By Muhammad Ali 5 minutes read

1. Introduction

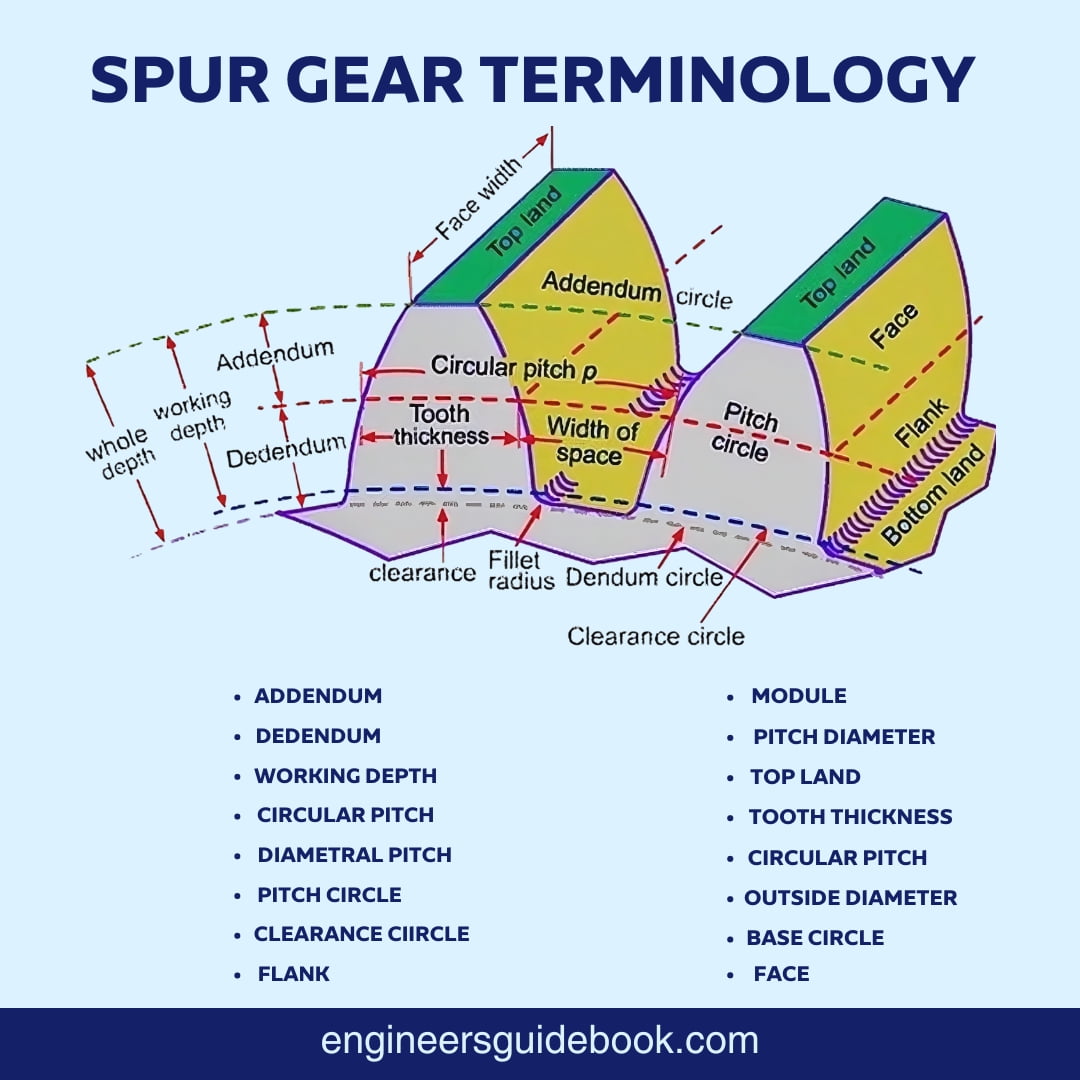
Spur gears are most commonly used gears in industry. You’ll find them in clocks, washing machines, electric screwdrivers, and even in industrial machinery. Their efficiency and simplicity make them ideal for applications where noise and vibration are not major concerns.

Because of their straight teeth, spur gears engage smoothly and create minimal friction, which means less wear and tear over time. They are the most common type of gears, featuring straight teeth cut parallel to the gear’s axis.

In this article, we will learn in detail about the critical terminology related to spur gears. We’ll also learn into the essential formulas needed for gear calculations. By the end of this guide, you’ll have a solid grasp of spur gear mechanics, making your designs more efficient and reliable.

2. Spur Gear Terminology

Addendum: The radial distance from the pitch circle to the top of the tooth.
Dedendum: The radial distance from the pitch circle to the bottom of the tooth.
Whole Depth: The total depth of the tooth space, equal to the sum of the addendum and dedendum.
Working Depth: The depth of the tooth in actual contact with the mating gear, equal to the sum of the addenda of both gears.
Clearance: The distance between the top of a tooth of one gear and the bottom of the mating space of the other gear.
Fillet Radius: The radius of the rounded corner at the base of the tooth.
Circular Pitch (p): The distance from one point on a gear tooth to the corresponding point on the next tooth, measured along the pitch circle.
Tooth Thickness: The thickness of a gear tooth measured along the pitch circle.
Width of Space: The width of the space between two adjacent teeth, measured along the pitch circle.
Face Width: The width of the tooth measured parallel to the gear axis.
Top Land: The flat surface at the top of a gear tooth.
Bottom Land: The flat surface at the bottom of the tooth space.
Addendum Circle: The circle that passes through the tops of the gear teeth.
Dedendum Circle: The circle that passes through the bottoms of the gear teeth.
Pitch Circle: The imaginary circle that rolls without slipping with a pitch circle of another gear.

Clearance Circle: The circle that is one clearance distance below the dedendum circle.
Face: The surface of the gear tooth between the pitch circle and the addendum circle.
Flank: The surface of the gear tooth between the pitch circle and the dedendum circle.
Gear Ratio: The ratio of the number of teeth on two meshing gears.
Pitch Diameter: The diameter of the pitch circle.
Module: The ratio of the pitch diameter to the number of teeth.
Base Circle: The circle from which the involute profile of the gear tooth is generated.
Base Circle Diameter: The diameter of the base circle.
Pressure Angle: The angle between the line of action and a line perpendicular to the pitch circle.
Contact Ratio: The average number of teeth in contact between two meshing gears.
Backlash: The clearance or play between mating gear teeth.
Helix Angle: The angle between the helix and an axial line on a helical gear.
Pitch Point: The point of tangency between the pitch circles of mating gears.
Root Diameter: The diameter of the circle that passes through the bottom of the tooth spaces.
Involute Profile: The curve generated by the end of a taut string as it is unwound from a circle, which defines the shape of the gear tooth.
Axial Pitch: The distance parallel to the gear axis between corresponding points on adjacent teeth.
Base Pitch: The distance along the base circle between corresponding points on adjacent teeth.
Root Clearance: The distance between the bottom of the tooth of one gear and the mating gear’s addendum circle.
Tip Relief: A slight modification of the tooth profile near the tip to reduce stress concentrations and noise.
Chordal Addendum: The height from the pitch circle to the top of the tooth measured along a chord.
Chordal Thickness: The thickness of a tooth measured along a chord passing through the pitch circle.
Runout: The total variation in the distance between the gear’s teeth and a fixed reference point, indicating eccentricity.
Lead: The distance a helical gear tooth advances along the gear axis for one complete rotation.
Tooth Face: The surface of a gear tooth that comes into contact with the tooth of another gear.
Back Angle: The angle between the backside of a gear tooth and a line perpendicular to the pitch circle.

3. Formulas for Spur Gears

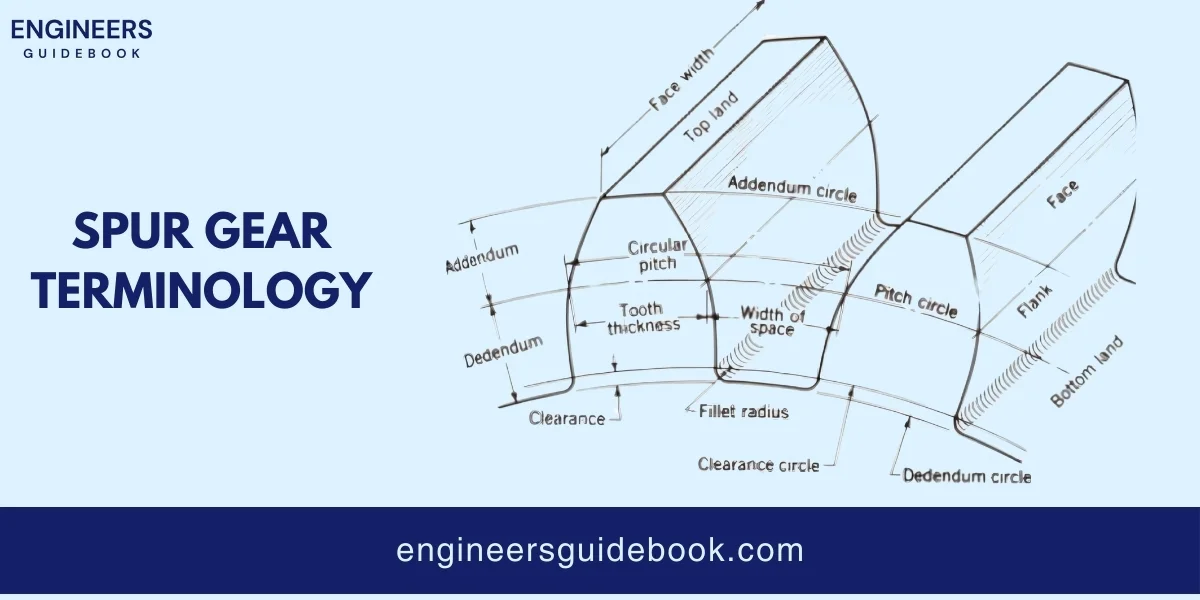
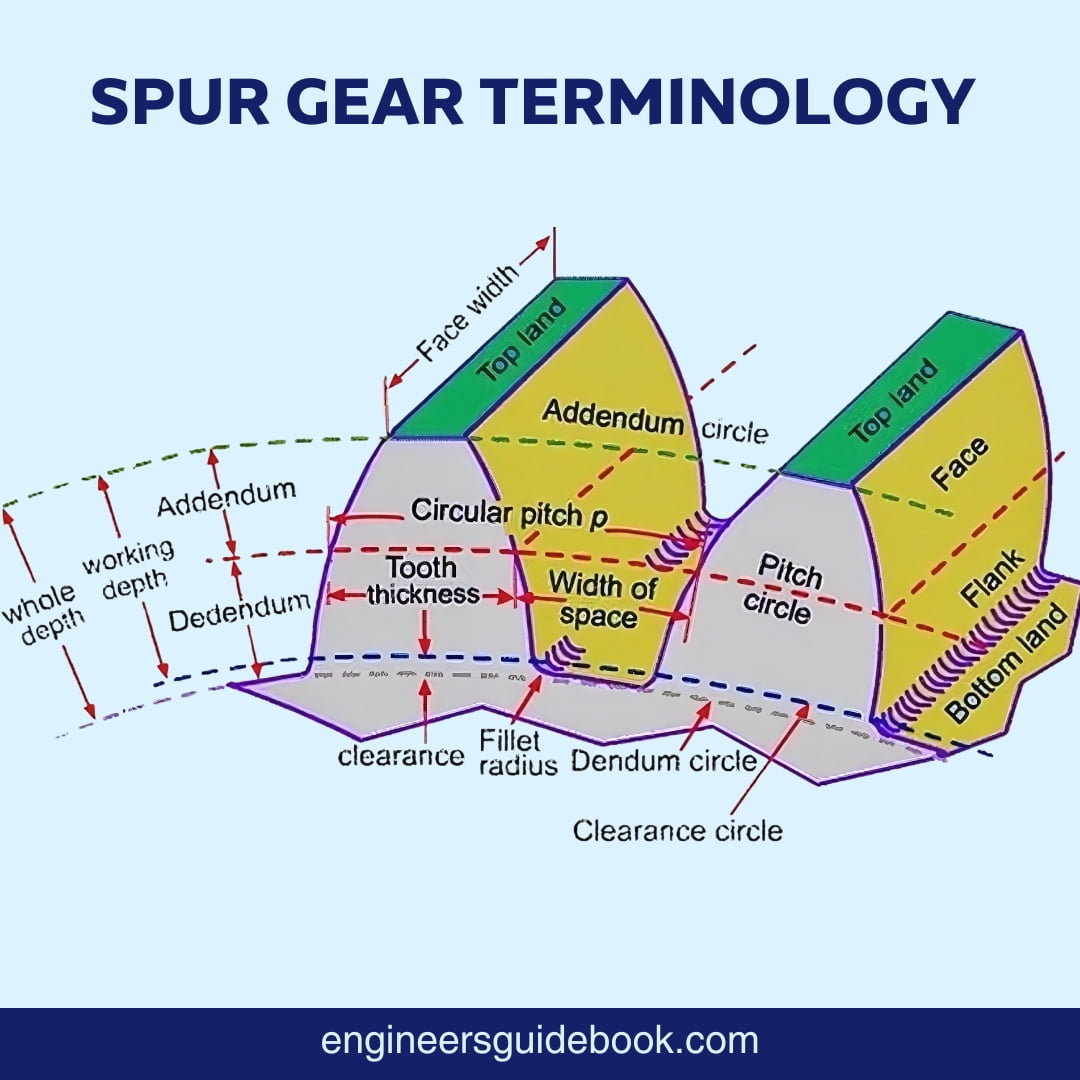
1. Gear Ratio Calculation

Gear Ratio = (No. of Teeth on Driven Gear) / (No. of Teeth on Driving Gear)

2. Diametral Pitch Calculation

Diametral Pitch (P) = Number of Teeth (N) / Pitch Diameter (D)

3. Pitch Diameter Calculation

Pitch Diameter (D) = Number of Teeth (N) / Diametral Pitch (P)

4. Circular Pitch Calculation

Circular Pitch (p) = (π × Pitch Diameter (D)) / Number of Teeth (N)

5. Module Calculation

Module (m) = Pitch Diameter (D) / Number of Teeth (N)

6. Addendum Calculation

Addendum (a) = 1 / Diametral Pitch (P)

7. Dedendum Calculation

Dedendum (d) = 1.25 / Diametral Pitch (P)

8. Tooth Thickness Calculation

Tooth Thickness = π / (2 × Diametral Pitch (P))

9. Base Circle Diameter Calculation

Base Circle Diameter (Db) = Pitch Diameter (D) × cos(Pressure Angle)

10. Contact Ratio Calculation

Contact Ratio = Arc of Action / Circular Pitch

4. Design Considerations for Spur Gears

Designing spur gears requires careful attention to several key factors to ensure efficiency and reliability.

Material Selection: Choose materials based on load capacity, wear resistance, noise reduction, and cost. Common options include steel, cast iron, bronze, and plastics.

Gear Tooth Profile: The involute profile ensures smooth operation. Tip relief and undercutting can prevent interference and reduce noise.

Load and Stress Analysis: Analyze static and dynamic loads. Use bending stress (Lewis formula) and contact stress (Hertzian theory) calculations. Incorporate safety factors to account for unexpected loads.

Gear Ratios and Speed: Calculate gear ratios to match application needs. Higher ratios increase torque but reduce speed.

Manufacturing Tolerances: Maintain precise tolerances for tooth thickness, pitch diameter, and runout. Implement strict quality control to ensure gears meet specifications.

Noise and Vibration Control: Use profile modifications, softer materials, and damping methods to minimize noise and vibration.

Backlash and Clearance: Adjust backlash to prevent binding and ensure smooth operation. Provide adequate clearance for lubrication and thermal expansion.

Conclusion

Understanding spur gears is essential for both engineering students and professionals. This guide has covered crucial terminology, key calculation formulas, and important design considerations.

By mastering terms like addendum, dedendum, pitch diameter, and module, and applying formulas for gear ratio, circular pitch, and tooth thickness, you can design gears that mesh correctly and operate efficiently. Practical considerations like material selection, load analysis, lubrication, and noise control ensure the reliability and longevity of gear systems. Real-world examples and step-by-step calculations illustrate the application of these principles.

With this knowledge, you are well-equipped to tackle gear design challenges, ensuring your gears perform optimally in various applications. Keep experimenting and applying these concepts to become proficient in gear engineering.

If you have any questions or need further resources, feel free to explore additional materials or seek professional guidance.

References

Gear Design Simplified by Franklin D. Jones and Henry H. Ryffel –
Mechanical Engineering Design by J.E. Shigley and C.R. Mischke
Gear Handbook: The Design, Manufacture, and Application of Gears edited by Darle W. Dudley
ANSI/AGMA 2001-D04: Fundamental Rating Factors and Calculation Methods for Involute Spur and Helical Gear Teeth –
Litvin, F. L., & Fuentes, A. (2004). Gear Geometry and Applied Theory.

FAQ'S

What is a spur gear?

A spur gear is a type of gear with straight teeth mounted on a parallel shaft, used to transmit motion and power efficiently between two rotating shafts.

How do spur gears work?

Spur gears work by meshing their teeth with those of another gear, transmitting torque and rotational motion from one gear to the other.

What are the applications of spur gears?

Spur gears are used in clocks, washing machines, conveyors, industrial machinery, and automotive transmissions.

What is the difference between spur gears and helical gears?

Spur gears have straight teeth; helical gears have angled teeth and offer smoother, quieter operation.

How do you calculate the gear ratio of spur gears?

The gear ratio is calculated by dividing the number of teeth on the driven gear by the number of teeth on the driving gear.

What materials are spur gears made of?

Spur gears can be made from steel, cast iron, bronze, and plastics, depending on the application requirements.

What is the pitch diameter of a gear?

The pitch diameter is the diameter of the pitch circle, which is an imaginary circle that rolls without slipping with another gear’s pitch circle.

How is the module of a spur gear defined?

The module is the ratio of the pitch diameter to the number of teeth, indicating the size of the gear teeth.

What are the advantages of using spur gears?

Advantages include simplicity in design, ease of manufacture, high efficiency, and the ability to transmit large amounts of power.

What is backlash in spur gears?

Backlash is the slight clearance or play between mating gear teeth, preventing gears from jamming and allowing for thermal expansion and lubrication.

What is addendum in spur gears?

Addendum is the radial distance from the pitch circle to the top of the tooth.

What is dedendum in spur gears?

Dedendum is the radial distance from the pitch circle to the bottom of the tooth.

How do you calculate circular pitch in spur gears?

Circular pitch is calculated as the circumference of the pitch circle divided by the number of teeth.

What is the function of the fillet radius in gear design?

The fillet radius is the rounded corner at the base of the tooth, reducing stress concentrations and increasing gear strength.

What is the role of lubrication in spur gear operation?

Lubrication reduces friction, wear, and overheating, ensuring smooth and efficient gear operation.

Spur Gear Terminology and Calculation